三角比の相互関係

【1】三角比の相互関係とは

ある角度\(\large{\theta}\) に対する三角比\(\large{\hspace{2pt}\sin \theta \hspace{2pt}}\)、\(\large{\cos \theta \hspace{2pt}}\)、\(\large{ \tan \theta \hspace{2pt}}\)の3つの値は、以下の関係式で結ばれます。

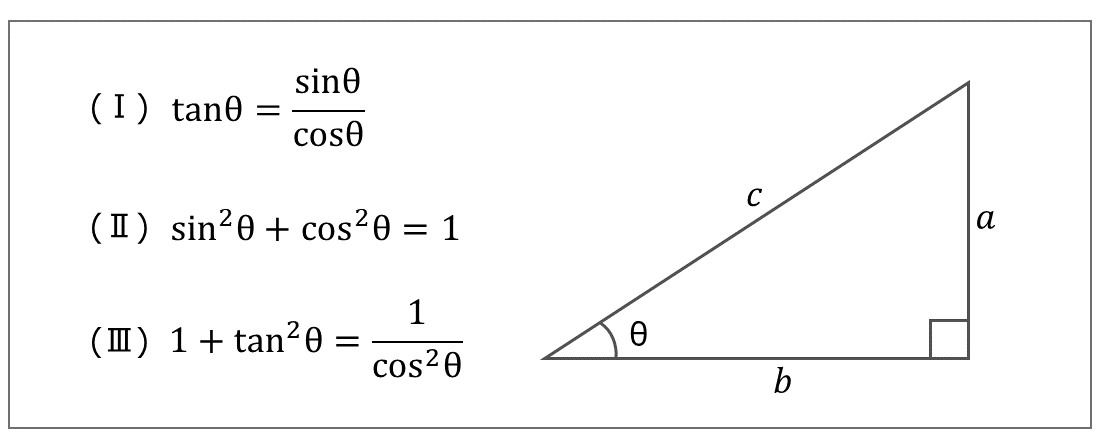
三角比の相互関係の式を使用することで、特定の角度の\(\large{\hspace{2pt} \sin \hspace{2pt}}\)、\(\large{\cos \hspace{2pt}}\)、\(\large{\tan \hspace{2pt}}\)のいずれか1つの値が分かれば、他の2つの値も求めることができます。

例えば、\(\large{0^\circ < \theta < 90^\circ}\) のある角度 \(\large{\theta}\) に対して \(\large{\sin \theta = \frac{4}{5}}\) であることが分かれば、(Ⅰ)～(Ⅲ)式から 角度\(\large{\hspace{2pt} \theta \hspace{2pt}}\)の値や、辺の長さ(\(\large{a,b,c}\))を用いなくても \( \large{\cos \theta = \frac{3}{5}}\)、\(\large{\tan \theta = \frac{4}{3}}\) と求めることができます。(練習問題(1))

本章では、上記の三角比の相互関係の式の導出を行います。

・三角比の相互関係の証明(Ⅰ)

まず、\(\displaystyle \large{\tan \theta = \frac{\sin \theta}{\cos \theta}}\)の式を証明します。

以下の図のような辺の長さ(\(\large{a}\),\(\large{b}\),\(\large{c}\))と角度 \(\large{\theta}\) を持った直角三角形を考えます。

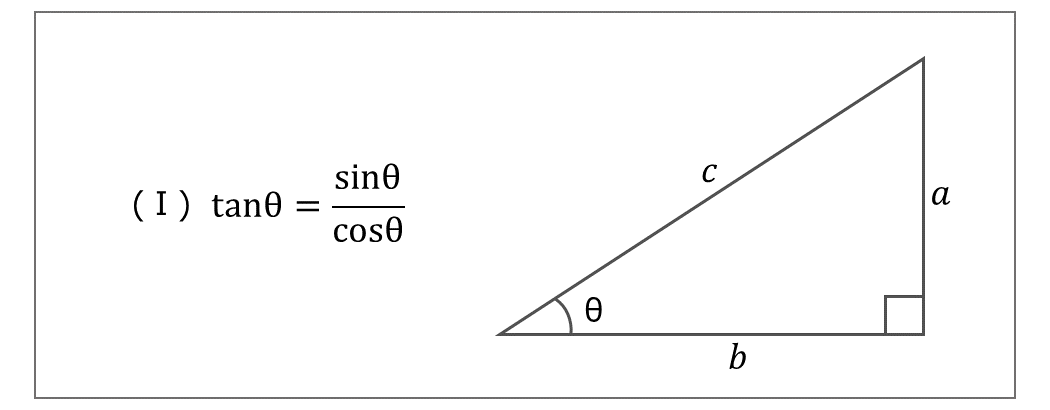
三角比の定義から、\(\large{\sin \theta}\) と \(\large{\cos \theta}\) は以下の式を満たします。 $$\large{\sin \theta = \frac{a}{c}}$$ $$\large{\cos \theta = \frac{b}{c}}$$

上式から、直角三角形の高さ\(\large{a}\)、底辺\(\large{b}\) は以下の式により表されます。 $$\large{a = c \sin \theta}$$ $$\large{b = c \cos \theta}$$

両式を割ると、以下の式を導くことができます。 $$\large{\frac{a}{b} = \frac{\sin \theta}{\cos \theta}}$$

ここで、三角比の定義から \(\displaystyle \large{\tan \theta = \frac{a}{b}}\) であるため、以下の式が成り立ちます。 $$\large{\tan \theta = \frac{\sin \theta}{\cos \theta}}$$

・三角比の相互関係の証明(Ⅱ)

次に、\(\large{\sin ^2 \theta + \cos^2 \theta =1}\) の式を導出します。

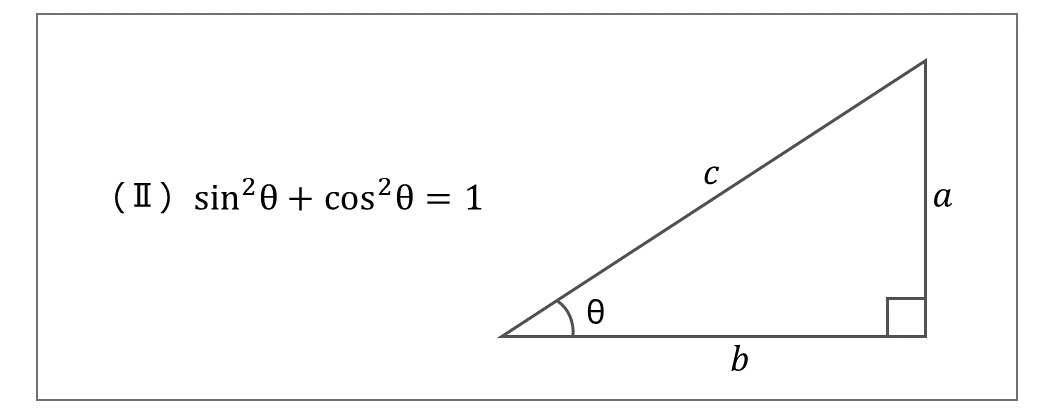
図の直角三角形に対して 三平方の定理を用いると、3辺に以下の関係が成り立ちます。 $$\large{a^2 + b^2 = c^2}$$

ここで、先述した関係式 \(\large{a = c \sin \theta}\)、\(\large{b = c \cos \theta}\) を上式に代入すると、以下のようになります。 $$\large{c^2 \sin ^2 \theta + c^2 \cos^2 \theta = c^2}$$ 両辺を \(\large{c^2}\) で割ると以下が得られます。(ただし、\(\large{c \neq 0}\)) $$\large{\sin ^2 \theta + \cos^2 \theta = 1}$$

・三角比の相互関係の証明(Ⅲ)

次に、\(\displaystyle \large{1 + \tan^2 \theta = \frac{1}{\cos^2 \theta}}\) の式を証明します。

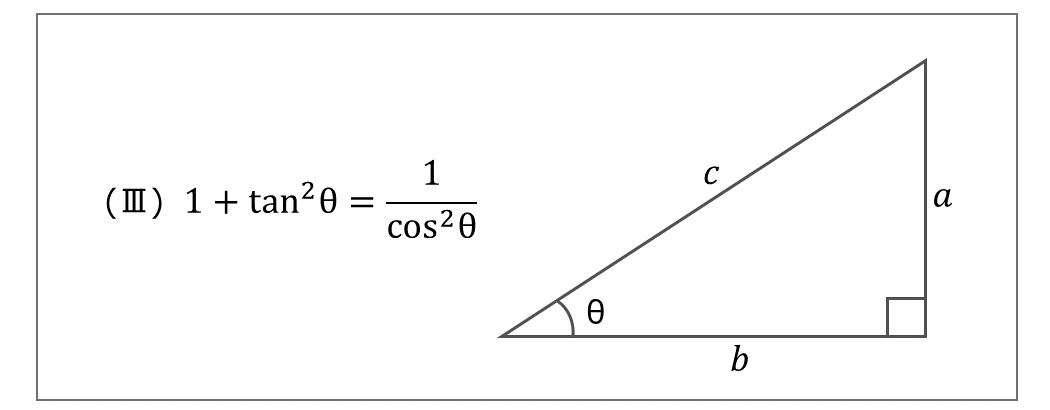
証明(Ⅱ)で導いた \(\large{\sin}\) と \(\large{\cos}\) の関係式(\(\large{\sin ^2 \theta + \cos^2 \theta = 1}\))の両辺を \(\large{\cos^2 \theta}\) で割ります。 $$\large{\frac{\sin ^2 \theta}{\cos^2 \theta} + 1 = \frac{1}{\cos^2 \theta}}$$

ここで、証明(Ⅰ)で導いた \(\displaystyle \large{\tan \theta = \frac{\sin \theta}{\cos \theta}}\) から、上式を変形し、以下の式が求められます。 $$\large{1 + \tan^2 \theta = \frac{1}{\cos^2 \theta}}$$

【2】三角比の相互関係の問題

三角比の相互関係の式に関連した例題を解説します。

問題(1) sinからcosとtanの導出

【解答と解説】
\(\large{\sin ^2 \theta + \cos^2 \theta = 1}\) から \(\large{\cos \theta}\) の値を求めると、以下のようになります。 \begin{eqnarray} \large \cos^2 \theta&\large =&\large 1-\sin ^2 \theta\\ \large &\large =&\large 1-{\left(\frac{4}{5}\right)}^2\\ \large &\large =&\large \frac{9}{25}\\ \end{eqnarray} したがって、 $$\large{\cos \theta = \frac{3}{5}}$$

また、\(\displaystyle \large{\tan \theta = \frac{\sin \theta}{\cos \theta}}\) の式から、\(\large{\tan \theta}\) を求めると以下のようなります。 $$\large{\tan \theta = \frac{\sin \theta}{\cos \theta}=\frac{4}{3}}$$

【補足】角度 \(\large{\theta}\) が鋭角 (\(\large{0^\circ < \theta < 90^\circ}\)) の場合は、\(\large{\sin \theta}\)、\(\large{\cos \theta}\)、\(\large{\tan \theta}\)は全て正の値で求めます。一方、鈍角 (\(\large{90^\circ < \theta < 180^\circ}\))では、\(\large{\sin \theta}\)が正の値で、\(\large{\cos \theta}\)と\(\large{\tan \theta}\)が負の値になります。

問題(2) tanからsinとcosの導出

【解答と解説】
\(\displaystyle \large{1 + \tan^2 \theta = \frac{1}{\cos^2 \theta}}\) の式から \(\large{\cos \theta}\) の値を求めると、以下のようになります。 \begin{eqnarray} \large \frac{1}{\cos^2 \theta}&\large =&\large 1+{\left(\frac{1}{2}\right)}^2\\ \large &\large =&\large \frac{5}{4}\\ \end{eqnarray} したがって、 $$\large{\cos \theta = \frac{2}{\sqrt{5}}}$$

また、\(\displaystyle \large{\tan \theta = \frac{\sin \theta}{\cos \theta}}\) の式から、\(\large{\sin \theta}\) を求めると以下のようなります。 $$\large{\sin \theta =\tan \theta \cos \theta=\frac{1}{\sqrt{5}}}$$

---