三次の三角関数を含む関数の最大値と最小値

【解答のポイント】
三角関数を含む関数の問題は、三角関数を異なる変数に置き換えることで、三次関数の微分の問題とすることができます。

本問では、\(\sin x = t\hspace{1pt}\)と置き換えて三次関数として最大値・最小値を求めます。

【解答】
まず、関数を\(\hspace{1pt}\sin x\hspace{1pt}\)のみの式に変形します。 $$\begin{aligned} y & = 4 \sin^3 x + 3 \cos^2 x\\[0.5em] & =4 \sin^3 x + 3 (1-\sin^2 x)\\[0.5em] & =4 \sin^3 x -3 \sin^2 x + 3\\[0.5em] \end{aligned}$$ 上式を\(\hspace{1pt}\sin x = t\hspace{1pt}\)と置き換えます。

このとき、\( 0 \leqq x \leqq \pi\hspace{2pt}\)であることから、\(\hspace{1pt}t\hspace{1pt}\)の範囲は $${ 0 \leqq t \leqq 1}$$ となります。

問題の関数を\(\hspace{1pt}t\hspace{1pt}\)に置き換えると $${ y = 4t^3 -3t^2 +3}$$ となります。

\(f(t)=4t^3 -3t^2 +3\hspace{1pt}\)とすると $${f'(t) = 12t^2 -6t }$$ となります。

\({f'(t)=0}\) を解くと $$\begin{aligned} 12t^2 -6t & =0\\[0.5em] 6t (2t -1) & =0\\[0.5em] \end{aligned}$$ よって\(\displaystyle\hspace{3pt}t = 0 , \frac{1}{2}\hspace{1pt}\)となります。

ここで、\(\displaystyle t =0 , \frac{1}{2}\hspace{1pt}\)の前後における \(\displaystyle{f'(t)}\) の符号の変化を調べます。

\({f'(t)=6t (2t -1) }\) より
　　\({\displaystyle t < 0}\)　　 のとき \({f'(t) > 0}\)
　　\({\displaystyle 0< t < \frac{1}{2}\hspace{2pt}}\) のとき \({f'(t) < 0}\)
　　\({\displaystyle t > \frac{1}{2}}\) 　　　のとき \({f'(t) > 0}\)
となります。

よって、関数\(\displaystyle {f(t)}\) は \({\displaystyle t = 0}\) で極大値、\({\displaystyle t=\frac{1}{2}}\) で極小値をとります。

以上から、増減表を作ると 以下のようになります。

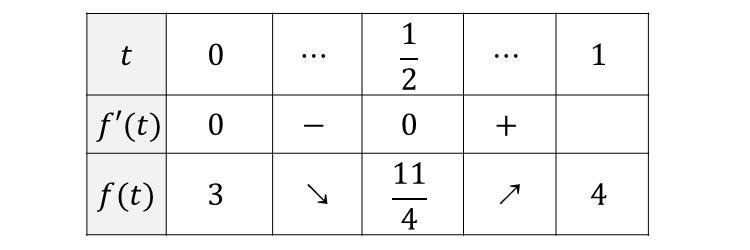
上記の増減表から、関数\(f(t)=4t^3 -3t^2 +3\hspace{1pt}\)\(\hspace{1pt}(0 \leqq t \leqq 1)\hspace{1pt}\) は
　\(\displaystyle t = \frac{1}{2}\hspace{1pt}\)で最小値\(\displaystyle\hspace{1pt}\frac{11}{4}\hspace{1pt}\)
　\(\displaystyle t = 1\hspace{1pt}\)で最大値\(\displaystyle\hspace{1pt}4\hspace{1pt}\)
となります。

ここで、\(\hspace{1pt}\sin x = t\hspace{1pt}\)\(\hspace{1pt}(0 \leqq x \leqq \pi)\hspace{1pt}\) であることから

　\(\displaystyle x = \frac{\pi}{6}, \frac{5}{6}\pi\hspace{1pt}\)で最小値\(\displaystyle\hspace{1pt}\frac{11}{4}\hspace{1pt}\)

　\(\displaystyle x = \frac{\pi}{2}\hspace{1pt}\)で最大値\(\displaystyle\hspace{1pt}4\hspace{1pt}\)

となります。